Research

WHY TOPOLOGY?

Felix Klein developed the idea that a geometry was defined in terms of its invariant properties. Euclidean geometry has dominated classical thinking, for it is the geometry constructed from the invariants of translation and rotations. That is, Euclidean geometry is the geometry of rigid body motion. Two obvious invariants are size and shape, but there are others. Such processes of translation and rotations are defined as diffeomorphisms.

Consider now an elastic object, say a rubber notebook sheet of paper with three holes in it. Certainly rigid motions of rotation and translation do not change the size and shape of the object. However bending of the sheet changes it shape, but not its size. Uniform expansion changes it size but not its shape. A general stretching can change both size and shape, but all of these things leave one property invariant. The number of holes does not change.

The number of holes, not their size or shape, is a topological property. A topological property is an invariant of a smooth deformation (no tearing apart or glueing together is permitted). Such processes are defined as homeomorphisms. The key idea is that such processes that preserve topological properties are continous, and have an inverse which is continuous.

The great bulk of classical physics is restricted to the study of geometric properties. Classic Tensor analysis assumes a domain that is covered by diffeomorphisms. Almost all of classical mechanics studies systems of invariant topology. In almost every case, the highly developed theories are REVERSIBLE. The parts of science that are controversial involve explanations of events where the topology of the initial state is not the same as the topology of the final state; where the process does not have a inverse. If the scientific objective is to understand the real irreversible world, then

WHAT ARE THE LAWS OF TOPOLOGICAL EVOLUTION?

Cartan’s theory of exterior differential forms can give part of the answer, for it appears that Cartan’s methods can be applied to problems of continuous topological evolution. Such problems do not have unique continuous inverses. Yet, by using the methods of functional substitution and the pullback (RETRODICTION) some headway can be made in the understanding of irreversible phenomena. For example, Cartan’s methods may be used to say something about the decay of turbulence, as a continuous irreversible process (think glueing together). The creation of turbulence (think discontinuous punctures or tearing into parts) is as of yet beyond current knowledge.

The idea is to learn about topology and topological properties, for when it is recognized that topology has changed during a process, then a signal has been given that such a process is irreversible in a thermodynamic sense. Irreversibility, up to now, has eluded physical theories, except in a statistical sense.

It’s going to be impossible to give you a full appreciation of what topology is, simply because it deals with mathematical ideas that are a lot more advanced than those you have seen so far.

but, here are some glimpses: think of geometric shapes like a circle, a square, and an annulus ( the region between two concentric circles) geometrically, they are very different. But there is something the circle and square have in common, a property not shared by the annulus. Start at a point inside the circle or square and draw any loop that remains in the circle or square and returns to the starting point. That loop will have the property that you can “shrink” it down to a point without any portion of the loop ever leaving the circle or square. But in the annulus, it is possible to draw a loop that surrounds the “hole” formed by the inner circle. It will be impossible to shrink that loop to a point without dragging part of it into the hole, and thus leaving the annulus. think about which geometric regions have this shrink-to-a-point property and which do not. You’ll soon discover that the distinction between the two types of regions has nothing to do with geometric properties like angles, areas, distances, etc. We call this loop property a topological property. So, loosely, the subject of topology studies a region by looking at the properites of that region that are independent of the geometric properties of the region. but it gets more abstract than that. typically, the regions being studied aren’t regions in the plane, except for textbook examples. They tend to be regions located in “topological spaces”, which are very abstract, often multidimensional, even infinite dimensional! As for applications, I think it is fair to say that most of the applications of topology are not directly to “real life.” On the other hand, topology provides mathematical tools that are useful to applied mathematicians and to theoretical physicists when they do their work. there is a theory among physicists that the universe is not just 3 dimensional, but that there are extra dimensions curled up in a complicated way on an infinitesimally tiny scale. Topology is used to describe this weird higher dimensional space we might be living in.

Topology is the study of which shapes are “topologically similar”.

Very generally speaking: Two shapes are “topologically similar” if you can match them by “stretching smoothly” without bunching or overlapping (called a 1-to-1 mapping) and whose boundaries (edges) are also the same shape (topologically). 1. Take a disk – a filled circle. In topology, a disk is the same as (topologically similar to) a “birthday hat” (an empty cone with no bottom). Why: First notice that edge/boundary of both the disk and the birthday hat are circles. Now think of the disk as a circle cut out of a balloon so that it is stretchy. If you are careful, you can glue it onto the birthday hat without overlapping or creasing by putting the center at the top point and pulling the boundary of the circle along the base of the hat. In this way each point of the balloon disk corresponds to a unique point on the birthday hat and vice-versa. 2. A disk is also topologically similar to a (filled) square or rectangle or triangle or indeed any shape cut out of cardboard with a single non-crossing, non-touching boundary, because angles do not “count” in topology. Again, one can stretch and glue the balloon disk onto a piece of cardboard cut into the shape. 3. A disk is NOT topologically similar to a (filled) figure 8 because there is no way to glue the balloon disk onto the figure 8 without “bunching” or overlapping of points around the crossing point.

————————————–… Topology also looks at “direction” or orientability.

1. Cut out two strips of paper. Draw a red line down the length of one side of each strip and a blue line down the other side. Mark arrows along the lines |—–>—–>—->—–>——>—–|. Now glue the ends of the first strip together to make a cylinder (red to red) and glue the ends of the other strip together to make a mobius band (red to blue). A cylinder is NOT topologically similar to a mobius band since a cylinder has two sides and a mobius band only one. There is no way to match them up. 2. A mobius band is “interesting” in topology because it is not “orientable” since as you go along the line it goes from —>—– to ———- . It constantly changes direction or “orientation”. 3. Notice that the cylinder band is orientable since as you go along a line on the cylinder the arrows always are in the same direction.

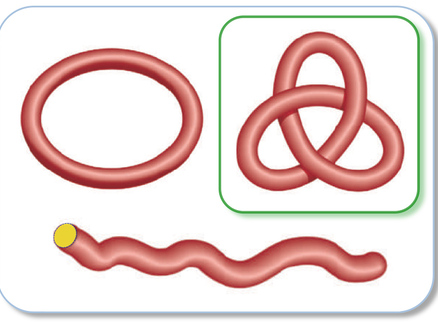
Topology (from the Greek τόπος, “place”, and λόγος, “study”) is the mathematical study of shapes and topological spaces. It is an area of mathematics concerned with the properties of space that are preserved under continuous deformations including stretching and bending, but not tearing or gluing. This includes such properties as connectedness, continuity and boundary.

Topology developed as a field of study out of geometry and set theory, through analysis of such concepts as space, dimension, and transformation. Such ideas go back to Leibniz, who in the 17th century envisioned the geometria situs (Greek-Latin for “geometry of place”) and analysis situs (Greek-Latin for “picking apart of place”). The term topology was introduced by Johann Benedict Listing in the 19th century, although it was not until the first decades of the 20th century that the idea of a topological space was developed. By the middle of the 20th century, topology had become a major branch of mathematics.

Topology began with the investigation of certain questions in geometry. Leonhard Euler‘s 1736 paper on the Seven Bridges of Königsberg^[1] is regarded as one of the first academic treatises in modern topology.

The term “Topologie” was introduced in German in 1847 by Johann Benedict Listing in Vorstudien zur Topologie,^[2] who had used the word for ten years in correspondence before its first appearance in print. The English form topology was first used in 1883 in Listing’s obituary in the journal Nature^[3] to distinguish “qualitative geometry from the ordinary geometry in which quantitative relations chiefly are treated”. The term topologist in the sense of a specialist in topology was used in 1905 in the magazine Spectator.^{[citation needed]} However, none of these uses corresponds exactly to the modern definition of topology.

Modern topology depends strongly on the ideas of set theory, developed by Georg Cantor in the later part of the 19th century. In addition to establishing the basic ideas of set theory, Cantor considered point sets in Euclidean space as part of his study of Fourier series.

Henri Poincaré published Analysis Situs in 1895,^[4] introducing the concepts of homotopy and homology, which are now considered part of algebraic topology.

Unifying the work on function spaces of Georg Cantor, Vito Volterra, Cesare Arzelà, Jacques Hadamard, Giulio Ascoli and others, Maurice Fréchet introduced the metric space in 1906.^[5] A metric space is now considered a special case of a general topological space. In 1914, Felix Hausdorff coined the term “topological space” and gave the definition for what is now called a Hausdorff space.^[6] Currently, a topological space is a slight generalization of Hausdorff spaces, given in 1922 by Kazimierz Kuratowski.^{[citation needed}

Topology can be formally defined as “the study of qualitative properties of certain objects (called topological spaces) that are invariant under a certain kind of transformation (called a continuous map), especially those properties that are invariant under a certain kind of transformation (called homeomorphism).”

Topology is also used to refer to a structure imposed upon a set X, a structure that essentially ‘characterizes’ the set X as a topological space by taking proper care of properties such as convergence, connectedness and continuity, upon transformation.

Topological spaces show up naturally in almost every branch of mathematics. This has made topology one of the great unifying ideas of mathematics.

The motivating insight behind topology is that some geometric problems depend not on the exact shape of the objects involved, but rather on the way they are put together. For example, the square and the circle have many properties in common: they are both one dimensional objects (from a topological point of view) and both separate the plane into two parts, the part inside and the part outside.

One of the first papers in topology was the demonstration, by Leonhard Euler, that it was impossible to find a route through the town of Königsberg (now Kaliningrad) that would cross each of its seven bridges exactly once. This result did not depend on the lengths of the bridges, nor on their distance from one another, but only on connectivity properties: which bridges are connected to which islands or riverbanks. This problem in introductory mathematics called Seven Bridges of Königsberg led to the branch of mathematics known as graph theory.

Similarly, the hairy ball theorem of algebraic topology says that “one cannot comb the hair flat on a hairy ball without creating a cowlick.” This fact is immediately convincing to most people, even though they might not recognize the more formal statement of the theorem, that there is no nonvanishing continuous tangent vector field on the sphere. As with the Bridges of Königsberg, the result does not depend on the shape of the sphere; it applies to any kind of smooth blob, as long as it has no holes.

To deal with these problems that do not rely on the exact shape of the objects, one must be clear about just what properties these problems do rely on. From this need arises the notion of homeomorphism. The impossibility of crossing each bridge just once applies to any arrangement of bridges homeomorphic to those in Königsberg, and the hairy ball theorem applies to any space homeomorphic to a sphere.

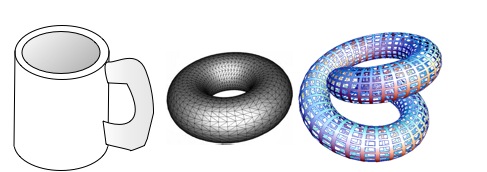
Intuitively, two spaces are homeomorphic if one can be deformed into the other without cutting or gluing. A traditional joke is that a topologist cannot distinguish a coffee mug from a doughnut, since a sufficiently pliable doughnut could be reshaped to a coffee cup by creating a dimple and progressively enlarging it, while shrinking the hole into a handle.

What is Topology and Why is it important?

https://www.youtube.com/watch?v=VudCtEtNXbI

• Ryszard Engelking, General Topology, Heldermann Verlag, Sigma Series in Pure Mathematics, December 1989, ISBN 3-88538-006-4.
• Bourbaki; Elements of Mathematics: General Topology, Addison–Wesley (1966).
• Breitenberger, E. (2006). “Johann Benedict Listing”. In James, I. M. History of Topology. North Holland. ISBN 978-0-444-82375-5.
• Kelley, John L. (1975). General Topology. Springer-Verlag. ISBN 0-387-90125-6.
• Brown, Ronald (2006). Topology and Groupoids. Booksurge. ISBN 1-4196-2722-8.  (Provides a well motivated, geometric account of general topology, and shows the use of groupoids in discussing van Kampen’s theorem, covering spaces, and orbit spaces.)
• Wacław Sierpiński, General Topology, Dover Publications, 2000, ISBN 0-486-41148-6
• Pickover, Clifford A. (2006). The Möbius Strip: Dr. August Möbius’s Marvelous Band in Mathematics, Games, Literature, Art, Technology, and Cosmology. Thunder’s Mouth Press. ISBN 1-56025-826-8.  (Provides a popular introduction to topology and geometry)
• Gemignani, Michael C. (1990) [1967], Elementary Topology (2nd ed.), Dover Publications Inc., ISBN 0-486-66522-4